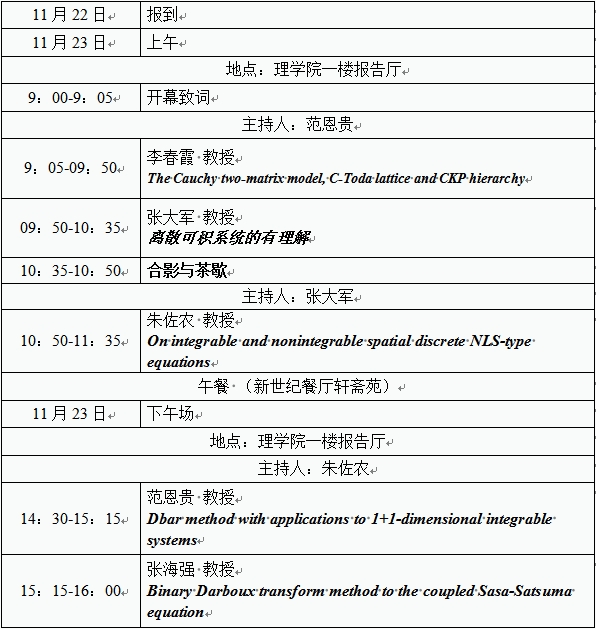
報告題目一🧝🏼:The Cauchy two-matrix model, C-Toda lattice and CKP hierarchy
報告時間:2019年11月23日09:05--09:50
報告地點:必一体育平台一樓報告廳
報告人:李春霞
報告人簡介: 李春霞🌦,中科院計算數學所博士👨🏼🚒,首都師範大學數學科學必一教授,主要從事數學物理等方面的研究🙅🏼。先後主持多項國家自然科學基金項目,北京市自然科學基金項目等🫃🏿。
報告摘要:In my talk, I will first give a brief review on some known results of the Cauchy bi-orthogonal polynomials. Starting from the symmetric reduction of Cauchy biorthogonal polynomials, we derive the Toda equation of CKP type (or the C-Toda lattice) as well as its Lax pair by introducing time flows. Then, matrix integral solutions to the C-Toda lattice are extended to give solutions to the CKP hierarchy which reveals the time-dependent partition function of the Cauchy two-matrix model is nothing but the Tau-function of the CKP hiearchy. At last, the connection between the Cauchy two-matrix model and Bures ensemble is established from the point of view of integrable systems.
報告題目二:離散可積系統的有理解
報告時間🏚🖱:2019年11月23日09:50—10:35
報告地點:必一体育平台一樓報告廳
報告人:張大軍
報告人簡介: 張大軍,上海大學數學系教授,博士生導師🤷🏽♂️。目前主要研究離散可積系統。SIDE (Symmetries and Integrability of Difference Equations) 會議指導委員會委員👨🏽🦲。2004年起先後作為國家公派留學生和訪問學者訪問芬蘭Turku大學物理系🧔🏽、英國Leeds大學非線性科學中心、York大學、Loughborough大學🥛、Glasgow大學、劍橋牛頓數學研究所🧑🏿✈️、美國Texas大學(Pan-American)等🍂🔨,並先後主持多項國家自然科學基金面上項目。
報告摘要:Hirota-Miwa equation (also known as Hirota’s equation/discrete AKP equation) is one of general 3D discrete integrable equations. Tau function of this equation admits an algebraic form, composed of polynomials of discrete independent coordinates. In this talk I will discuss properties of such a tau function and its applications in constructing rational solutions of integrable quadrilateral equations (such as the Nijhoff-Quispel-Capel equation, equations in the Adler-Bobenko-Suris (ABS) list and some multi-quadratic ABS equations). The tau function obeys a bilinear superposition formula, which provides generalized Burchnall-Chaundy polynomials.
報告題目三:On integrable and nonintegrable spatial discrete NLS-type equations
報告時間:2019年11月23日10:50—11▫️♓️:35
報告地點🙅🏼♂️:必一体育平台一樓報告廳
報告人:朱佐農
報告人簡介: 朱佐農👩🏽🎤,上海交通大學數學科學必一教授,博士生導師。學術研究領域是數學物理🪆,研究方向是孤立子和可積系統理論,在連續和離散的可積系統的研究上取得若幹重要進展👩🚒。先後主持國家自然科學基金項目、上海市浦江人才計劃項目和教育部留學回國人員基金項目🙍🏽♀️。
報告摘要:In this talk, we will focus on the topic on integrable and nonintegrable spatial discrete nonlinear Schrodinger-type equations, including integrable and nonintegrable spatial discrete NLS equations, integrable and nonintegrable spatial discrete Hirota equations, and integrable and nonintegrable spatial discrete nonlocal NLS equations. This talk is based on the joint works with L.Y. Ma, C.Q. Song, J.L. Ji and Z.W. Xu.
報告題目四:Dbar method with applications to 1+1-dimensional integrable systems
報告時間👄:2019年11月23日14:30—15🕵️♂️🛼:15
報告地點:必一体育平台一樓報告廳
報告人:範恩貴
報告人簡介: 範恩貴,復旦大學教授🏄🏻👏🏽、博士生導師,曾獲教育部自然科學二等獎、上海市自然科學二等獎👱🏽、國際“湯姆森路透卓越研究獎”🐦⬛、復旦大學谷超豪數學獎🧜🏿♂️;主要研究方向是孤立子理論🤸♀️、可積系統🧢、Riemann-Hilbert問題、正交多項式和隨機矩陣理論;近年來,連續兩屆為國家“973”課題成員🌮,並主持國家自然科學基金📮、上海曙光計劃🎎、上海曙光計劃跟蹤課題等多項研究課題。
報告摘要🔳:In this talk, we first introduce short history of inverse scattering theory, then compare difference and connections among inverse scattering transformation, Riemann-Hilbert approach and dbar method. At last, we provide some applications in 1+1-dimensional integrable systems.
報告題目五:Binary Darboux transform method to the coupled Sasa-Satsuma equation
報告時間:2019年11月23日15:15—16👩👩👦👦:00
報告地點:必一体育平台一樓報告廳
報告人✊🏽:張海強
報告人簡介: 張海強🏪,必一体育滬江學者教授。
報告摘要:The binary Darboux transformation method is applied to the coupled Sasa-Satsuma equations, which can be used to describe the propagation dynamics of femtosecond vector solitons in the birefringent fibers with third-order dispersion, self-steepening, and stimulated Raman scattering higher-order effects. An N-fold iterative formula of the resulting binary Darboux transformation is presented in terms of the quasideterminants. Via the simplest case of this formula, a few of illustrative explicit solutions to the coupled Sasa-Satsuma equations are generated from vanishing and non-vanishing backgrounds, which include the breathers, single- and double-hump bright vector solitons, and anti-dark vector solitons.

